Difference between revisions of "Problems on Deligne-Mumford spaces"
Natebottman (Talk | contribs) |
Natebottman (Talk | contribs) |
||
| Line 20: | Line 20: | ||
...and, to the operadically initiated (or willing to dig around a little at [[http://ncatlab.org]]): show that the collection <math>(K_d)_{d\geq 2}</math> can be given the structure of an '''operad''' (which is to say that for every <math>d, e \geq 2</math> and <math>1 \leq i \leq d</math> there is a composition operation <math>\circ_i\colon K_d \times K_e \to K_{d+e-1}</math> which splices <math>T_e \in K_e</math> onto <math>T_d \in K_d</math> by identifying the outgoing edge of <math>T_e</math> with the <math>i</math>-th incoming edge of <math>T_d</math>, and that these operations satisfy some coherence conditions). | ...and, to the operadically initiated (or willing to dig around a little at [[http://ncatlab.org]]): show that the collection <math>(K_d)_{d\geq 2}</math> can be given the structure of an '''operad''' (which is to say that for every <math>d, e \geq 2</math> and <math>1 \leq i \leq d</math> there is a composition operation <math>\circ_i\colon K_d \times K_e \to K_{d+e-1}</math> which splices <math>T_e \in K_e</math> onto <math>T_d \in K_d</math> by identifying the outgoing edge of <math>T_e</math> with the <math>i</math>-th incoming edge of <math>T_d</math>, and that these operations satisfy some coherence conditions). | ||
| − | Next, show that algebras / categories | + | Next, show that algebras / categories over the operad <math>(C_*(K_d))_{d\geq2}</math> of cellular chains on <math>K_d</math> are the same thing as <math>A_\infty</math> algebra / categories. |
Revision as of 16:09, 26 May 2017
2-, 3-dimensional associahedra
As described in Deligne-Mumford space, for any 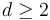 , the associahedron
, the associahedron 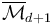 is a
is a  -dimensional manifold with boundary and corners which parametrizes nodal trees of disks with
-dimensional manifold with boundary and corners which parametrizes nodal trees of disks with  marked points, one of them distinguished (we think of the
marked points, one of them distinguished (we think of the 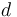 undistinguished resp. 1 distinguished marked points as "input" resp. "output" marked points).
As shown in [Auroux, Ex. 2.6],
undistinguished resp. 1 distinguished marked points as "input" resp. "output" marked points).
As shown in [Auroux, Ex. 2.6], 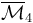 is homeomorphic to a closed interval, with one endpoint corresponding to a collision of the first two inputs
is homeomorphic to a closed interval, with one endpoint corresponding to a collision of the first two inputs 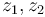 and the other corresponding to a collision of
and the other corresponding to a collision of 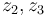 .
Work out which polygon/polyhedron
.
Work out which polygon/polyhedron  are equal to.
(Keep in mind that when
are equal to.
(Keep in mind that when  marked points collide simultaneously, there is a continuous family of ways that this collision can take place.)
marked points collide simultaneously, there is a continuous family of ways that this collision can take place.)
poset underlying associahedra
The associahedron 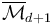 can be given the structure of a stratified space, where the underlying poset is called
can be given the structure of a stratified space, where the underlying poset is called 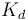 and consists of stable rooted ribbon trees with
and consists of stable rooted ribbon trees with 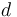 leaves.
Similarly to the setup in Moduli spaces of pseudoholomorphic polygons, a stable rooted ribbon tree is a tree
leaves.
Similarly to the setup in Moduli spaces of pseudoholomorphic polygons, a stable rooted ribbon tree is a tree 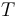 satisfying these properties:
satisfying these properties:
-
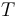 has
has 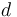 leaves and 1 root (in our terminology, the root has valence 1 but is not counted as a leaf);
leaves and 1 root (in our terminology, the root has valence 1 but is not counted as a leaf); -
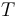 is stable, i.e. every main vertex (=neither a leaf nor the root) has valence at least 3;
is stable, i.e. every main vertex (=neither a leaf nor the root) has valence at least 3; -
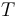 is a ribbon tree, i.e. the edges incident to any vertex are equipped with a cyclic ordering.
is a ribbon tree, i.e. the edges incident to any vertex are equipped with a cyclic ordering.
Write the closure of the stratum corresponding to 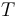 as a product of lower-dimensional
as a product of lower-dimensional 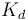 's.
Which tree corresponds to the top stratum of
's.
Which tree corresponds to the top stratum of 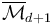 ?
To the codimension-1 strata of
?
To the codimension-1 strata of 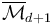 ?
?
...and, to the operadically initiated (or willing to dig around a little at [[1]]): show that the collection 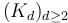 can be given the structure of an operad (which is to say that for every
can be given the structure of an operad (which is to say that for every 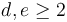 and
and 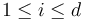 there is a composition operation
there is a composition operation  which splices
which splices 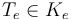 onto
onto  by identifying the outgoing edge of
by identifying the outgoing edge of 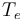 with the
with the  -th incoming edge of
-th incoming edge of 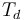 , and that these operations satisfy some coherence conditions).
Next, show that algebras / categories over the operad
, and that these operations satisfy some coherence conditions).
Next, show that algebras / categories over the operad 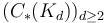 of cellular chains on
of cellular chains on 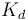 are the same thing as
are the same thing as 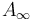 algebra / categories.
algebra / categories.