Difference between revisions of "Deligne-Mumford space"

From Polyfolds.org
Jump to: navigation, search
m
Line 9: Line 9:
 
</math></center>
 
</math></center>
 
can be compactified to form the [[Deligne-Mumford space]] <math>\overline\mathcal{M}_{d+1}</math>, whose boundary and corner strata can be represented by trees of polygonal domains <math>(\Sigma_v)_{v\in V}</math> with each edge <math>e=(v,w)</math> represented by two punctures <math>z^-_e\in \Sigma_v</math> and <math>z^+_e\in\Sigma_w</math>. The ''thin'' neighbourhoods of these punctures are biholomorphic to half-strips, and then a neighbourhood of a tree of polygonal domains is obtained by gluing the domains together at the pairs of strip-like ends represented by the edges.
 
can be compactified to form the [[Deligne-Mumford space]] <math>\overline\mathcal{M}_{d+1}</math>, whose boundary and corner strata can be represented by trees of polygonal domains <math>(\Sigma_v)_{v\in V}</math> with each edge <math>e=(v,w)</math> represented by two punctures <math>z^-_e\in \Sigma_v</math> and <math>z^+_e\in\Sigma_w</math>. The ''thin'' neighbourhoods of these punctures are biholomorphic to half-strips, and then a neighbourhood of a tree of polygonal domains is obtained by gluing the domains together at the pairs of strip-like ends represented by the edges.
 +
 +
 +
The space of stable rooted metric ribbon trees, as discussed in [[https://books.google.com/books/about/Homotopy_Invariant_Algebraic_Structures.html?id=Sz5yQwAACAAJ BV]],  is another topological representation of the (compactified) [[Deligne Mumford space]] <math>\overline\mathcal{M}_{d+1}</math>.

Revision as of 13:53, 24 May 2017

table of contents

TODO: Expand on this copy of a sketch in the moduli space construction

For 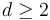d\geq 2, the moduli space of domains

{\mathcal {M}}_{{d+1}}:={\frac {{\bigl \{}\Sigma _{{\underline {z}}}\,{\big |}\,\underline {z}=\{z_{0},\ldots ,z_{d}\}\in \partial D\;{\text{pairwise disjoint}}{\bigr \}}}{\Sigma _{{\underline {z}}}\sim \Sigma _{{\underline {z}'}}\;{\text{iff}}\;\exists \psi :\Sigma _{{\underline {z}}}\to \Sigma _{{\underline {z}'}},\;\psi ^{*}i=i}}

can be compactified to form the Deligne-Mumford space 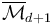\overline {\mathcal {M}}_{{d+1}}, whose boundary and corner strata can be represented by trees of polygonal domains (\Sigma _{v})_{{v\in V}} with each edge e=(v,w) represented by two punctures z_{e}^{-}\in \Sigma _{v} and z_{e}^{+}\in \Sigma _{w}. The thin neighbourhoods of these punctures are biholomorphic to half-strips, and then a neighbourhood of a tree of polygonal domains is obtained by gluing the domains together at the pairs of strip-like ends represented by the edges.


The space of stable rooted metric ribbon trees, as discussed in [BV], is another topological representation of the (compactified) Deligne Mumford space 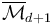\overline {\mathcal {M}}_{{d+1}}.

Retrieved from "http://www.polyfolds.org/index.php?title=Deligne-Mumford_space&oldid=290"