Difference between revisions of "Problems on Deligne-Mumford spaces"
From Polyfolds.org
Natebottman (Talk | contribs) |
Natebottman (Talk | contribs) |
||
| Line 6: | Line 6: | ||
As shown in [Auroux, Ex. 2.6], <math>\overline\mathcal{M}_4</math> is homeomorphic to a closed interval, with one endpoint corresponding to a collision of the first two inputs <math>z_1, z_2</math> and the other corresponding to a collision of <math>z_2, z_3</math>. | As shown in [Auroux, Ex. 2.6], <math>\overline\mathcal{M}_4</math> is homeomorphic to a closed interval, with one endpoint corresponding to a collision of the first two inputs <math>z_1, z_2</math> and the other corresponding to a collision of <math>z_2, z_3</math>. | ||
Work out which polygon/polyhedron <math>\overline\mathcal{M}_5, \overline\mathcal{M}_6</math> are equal to. | Work out which polygon/polyhedron <math>\overline\mathcal{M}_5, \overline\mathcal{M}_6</math> are equal to. | ||
| − | (Keep in mind that when <math>\geq 3</math> marked points collide simultaneously, there is a continuous family | + | (Keep in mind that when <math>\geq 3</math> marked points collide simultaneously, there is a continuous family of ways that this collision can take place.) |
Revision as of 14:07, 26 May 2017
poset underlying associahedra
As described in Deligne-Mumford space, for any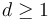 
, 
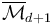is a 
-dimensional manifold with boundary and corners which parametrizes nodal trees of disks with 
marked points, one of them distinguished (we think of the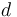 
undistinguished resp. 1 distinguished marked points as "input" resp. "output" marked points).
As shown in [Auroux, Ex. 2.6],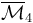 
is homeomorphic to a closed interval, with one endpoint corresponding to a collision of the first two inputs 
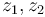and the other corresponding to a collision of 
.
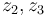Work out which polygon/polyhedron 
are equal to.
(Keep in mind that when 
marked points collide simultaneously, there is a continuous family of ways that this collision can take place.)
