Problems on Deligne-Mumford spaces
These problems deal with Deligne-Mumford spaces, by which we mean the moduli spaces of domains relevant for our construction of the Fukaya category. The first problem forms the warm-up portion: you should make sure you understand how to do this one before Tuesday morning. The remaining two problems form the further fun section: useful for deeper understanding, but not essential for following the thread of the lectures.
Down and dirty with low-dimensional associahedra
Using the notation of Deligne-Mumford space, for any 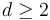 , the associahedron
, the associahedron 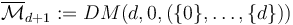 is a
is a  -dimensional manifold with boundary and corners which parametrizes nodal trees of disks with
-dimensional manifold with boundary and corners which parametrizes nodal trees of disks with  marked points, one of them distinguished (we think of the
marked points, one of them distinguished (we think of the 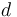 undistinguished resp. 1 distinguished marked points as "input" resp. "output" marked points).
The associahedra are one of the Deligne-Mumford spaces we will use during the summer school, corresponding to the situation where all the Lagrangians labeling the boundary segments are distinct.
undistinguished resp. 1 distinguished marked points as "input" resp. "output" marked points).
The associahedra are one of the Deligne-Mumford spaces we will use during the summer school, corresponding to the situation where all the Lagrangians labeling the boundary segments are distinct.
(a) As shown in [Auroux, Ex. 2.6 [[1]]], 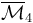 is homeomorphic to a closed interval, with one endpoint corresponding to a collision of the first two inputs
is homeomorphic to a closed interval, with one endpoint corresponding to a collision of the first two inputs 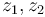 and the other corresponding to a collision of
and the other corresponding to a collision of 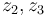 .
Moving up a dimension,
.
Moving up a dimension, 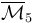 is a pentagon; it can be identified with the central pentagon in the depiction of
is a pentagon; it can be identified with the central pentagon in the depiction of  in Deligne-Mumford space.
Which polyhedron is
in Deligne-Mumford space.
Which polyhedron is 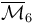 equal to?
(A good way to get started on this problem is to list the codimension-1 strata.)
equal to?
(A good way to get started on this problem is to list the codimension-1 strata.)
(b) Using the manifold-with-corners structure of the associahedra constructed in Deligne-Mumford space, observe that  can be covered by three charts:
can be covered by three charts:
- boundary charts centered respectively at the two points in
 (the domains of these charts are of the form
(the domains of these charts are of the form 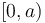 , and a choice of a point in this interval tells us how much to smooth the node);
, and a choice of a point in this interval tells us how much to smooth the node); - an interior chart (which we produce by fixing the positions of three of the marked points, and varying the positive of the fourth).
Explicitly work out these charts, and the transition maps amongst them.
The poset indexing the strata of the associahedra
The associahedron 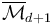 can be given the structure of a stratified space, where the underlying poset is called
can be given the structure of a stratified space, where the underlying poset is called 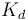 and consists of stable rooted ribbon trees with
and consists of stable rooted ribbon trees with 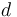 leaves.
Similarly to the setup in Moduli spaces of pseudoholomorphic polygons, a stable rooted ribbon tree is a tree
leaves.
Similarly to the setup in Moduli spaces of pseudoholomorphic polygons, a stable rooted ribbon tree is a tree 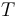 satisfying these properties:
satisfying these properties:
-
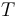 has
has 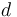 leaves and 1 root (in our terminology, the root has valence 1 but is not counted as a leaf);
leaves and 1 root (in our terminology, the root has valence 1 but is not counted as a leaf); -
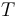 is stable, i.e. every main vertex (=neither a leaf nor the root) has valence at least 3;
is stable, i.e. every main vertex (=neither a leaf nor the root) has valence at least 3; -
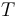 is a ribbon tree, i.e. the edges incident to any vertex are equipped with a cyclic ordering.
is a ribbon tree, i.e. the edges incident to any vertex are equipped with a cyclic ordering.
To define the partial order, we declare 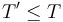 if we can contract some of the interior edges in
if we can contract some of the interior edges in 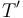 to get
to get 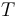 ; we declare that
; we declare that 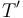 is in the closure of
is in the closure of 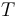 if
if 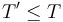 .
Write the closure of the stratum corresponding to
.
Write the closure of the stratum corresponding to 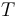 as a product of lower-dimensional
as a product of lower-dimensional 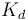 's.
Which tree corresponds to the top stratum of
's.
Which tree corresponds to the top stratum of 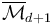 ?
To the codimension-1 strata of
?
To the codimension-1 strata of 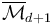 ?
?
...and, to the operadically initiated (or willing to dig around a little at [[2]]): show that the collection 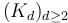 can be given the structure of an operad (which is to say that for every
can be given the structure of an operad (which is to say that for every 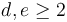 and
and 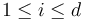 there is a composition operation
there is a composition operation  which splices
which splices 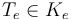 onto
onto  by identifying the outgoing edge of
by identifying the outgoing edge of 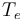 with the
with the  -th incoming edge of
-th incoming edge of 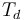 , and that these operations satisfy some coherence conditions).
Next, show that algebras / categories over the operad
, and that these operations satisfy some coherence conditions).
Next, show that algebras / categories over the operad 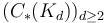 of cellular chains on
of cellular chains on 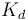 are the same thing as
are the same thing as 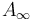 algebra / categories.
algebra / categories.
More low-dimensional examples of Deligne-Mumford spaces
(a) Explicitly work out the 3-dimensional Deligne-Mumford space 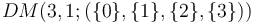 .
.
(b) For 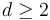 define
define 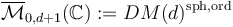 .
That is, if we define
.
That is, if we define

where two configurations are identified if one can be taken to the other by a Moebius transformation, then 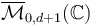 is the compactification defined by including stable trees of spheres, where every sphere has at least 3 special (marked/nodal) points and any neighboring pair of spheres is attached at a pair of points.
(A detailed construction of
is the compactification defined by including stable trees of spheres, where every sphere has at least 3 special (marked/nodal) points and any neighboring pair of spheres is attached at a pair of points.
(A detailed construction of 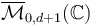 can be found in [big McDuff-Salamon, App. D].)
Make the identifications (don't worry too much about rigor)
can be found in [big McDuff-Salamon, App. D].)
Make the identifications (don't worry too much about rigor) 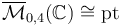 ,
, 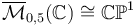 , and
, and 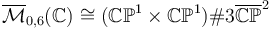 .
.