Moduli spaces of pseudoholomorphic polygons

To construct the moduli spaces from which the composition maps are defined we fix an auxiliary almost complex structure $J:TM\to TM$ which is compatible with the symplectic structure in the sense that $\omega (\cdot ,J\cdot )$ defines a metric on $M$. (Unless otherwise specified, we will use this metric in all following constructions.) Then given Lagrangians $L_{0},\ldots ,L_{d}\subset M$ and generators $x_{0}\in {\text{Crit}}(L_{0},L_{d}),x_{1}\in {\text{Crit}}(L_{0},L_{1}),\ldots ,x_{d}\in {\text{Crit}}(L_{{d-1}},L_{d})$ of their morphism spaces, we need to specify the moduli space $\overline {\mathcal {M}}(x_{0};x_{1},\ldots ,x_{d})$. We will do this below by combining two special cases.

Disk trees for a fixed Lagrangian

If the Lagrangians are all the same, $L_{0}=L_{1}=\ldots =L_{d}=:L$, then our construction is based on pseudoholomorphic disks

$u:D\to M,\qquad u(\partial D)\subset L,\qquad \overline \partial _{J}u=0.$

For a sequence of such maps, energy concentration at a boundary point is usually captured in terms of a disk bubble attached via a boundary node. This yields to a compactification of the moduli space of pseudoholomorphic disks modulo reparametrization that is given by adding boundary strata consisting of fiber products of moduli spaces of disks. One could - as proposed by Fukaya et al - regularize such a space and then interpret disk bubbling as contribution to $\widehat \mu \circ \widehat \mu$ - where the composition is via a push-pull construction on some space of differential chains on the Lagrangian. However, such push-pull constructions require transversality of evaluation maps to the differential chains, so that a rigorous construction of the 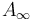$A_{\infty }$-structure in this setting would require a complicated infinite iteration.

We will resolve this issue as in [JL] by following another earlier proposal by Fukaya-Oh to allow disks to flow apart along a Morse trajectory. Here we work throughout with the Morse function 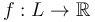$f:L\to \mathbb{R}$ chosen in the setup of the morphism space ${\text{Hom}}(L,L)=\textstyle \sum _{{x\in {\text{Crit}}(f)}}\Lambda x$. We also choose a metric on $L$ so that the gradient vector field $\nabla f$ satisfies the Morse-Smale conditions and an additional technical assumption in [1] which guarantees a smooth manifold-with-boundary-and-corners structure on the compactified Morse trajectory spaces $\overline {\mathcal {M}}(L,L),\overline {\mathcal {M}}(p^{-},L),\overline {\mathcal {M}}(L,p^{+}),\overline {\mathcal {M}}(p^{-},p^{+})$ for $p^{\pm }\in {\text{Crit}}(f)$.

[JL]

$todo$

TODO: compact if J with no spheres ... otherwise add spheres as below

Pseudoholomorphic polygons for pairwise transverse Lagrangians

If each consecutive pair of Lagrangians is transverse, i.e. $L_{0}\pitchfork L_{1},L_{1}\pitchfork L_{2},\ldots ,L_{{d-1}}\pitchfork L_{d},L_{d}\pitchfork L_{0}$, then our construction is based on pseudoholomorphic polygons

$u:\Sigma \to M,\qquad u((\partial \Sigma )_{i})\subset L_{i},\qquad \overline \partial _{J}u=0,$

where $\Sigma =D\setminus \{z_{0},\ldots ,z_{d}\}$ is a disk with $d+1$ boundary punctures in counter-clockwise order $z_{0},\ldots ,z_{d}\subset \partial D$, and $(\partial \Sigma )_{i}$ denotes the boundary component between $z_{i},z_{{i+1}}$ (resp. between $z_{{d}},z_{0}$ for i=d).

General moduli space of pseudoholomorphic polygons

Make up for differences in Hamiltonian symplectomorphisms applied to each Lagrangian by a domain-dependent Hamiltonian perturbation to the Cauchy-Riemann equation

Finally, the symplectic area function $\omega :\overline {\mathcal {M}}(x_{0};x_{1},\ldots ,x_{d})\to \mathbb{R}$ in each case is given by TODO