Scale calculus problems

Scale calculus was developed to make reparametrization actions on function spaces smooth. All the key issues and ideas can already be seen at the example of $S^{1}=\mathbb{R} /\mathbb{Z }$ acting on a space of nonconstant functions on $S^{1}$, for example the shift action

$\sigma :S^{1}\times {\mathcal {C}}_{{{\rm {id}}}}^{1}\;\to \;{\mathcal {C}}_{{{\rm {id}}}}^{1},\quad (\tau ,u)\mapsto u(\tau +\cdot )$

${\text{on}}\qquad \qquad \qquad \qquad {\mathcal {C}}_{{{\rm {id}}}}^{1}:=\{u:S^{1}\to S^{1}\;|\;u\in {\mathcal {C}}^{1}\;{\text{and homotopic to the identity}}\}.\qquad \qquad \qquad \qquad .$

The purpose of the following exercises is to give the quotient of S^1-valued functions homotopic to the identity, modulo reparametrization by shifts, ${\mathcal {B}}:={\mathcal {C}}_{{{\rm {id}}}}^{1}/S^{1}$ the structure of a scale-smooth manifold ... and to see that classical Banach space topologies fail at giving it a differentiable Banach manifold structure.

differentiability of shift map

Compute the directional derivatives of the shift map $\sigma$, first at $(\tau ,u)=(0,0)$, then at $(\tau ,u)\in S^{1}\times {\mathcal {C}}_{{{\rm {id}}}}^{2}$.

Next, understand how to identify $T{\mathcal {C}}_{{{\rm {id}}}}^{1}\simeq {\mathcal {C}}^{1}(S^{1},\mathbb{R} )$, and write down a conjectural formula for the differential $d\sigma :\mathbb{R} \times {\mathcal {C}}^{1}(S^{1},\mathbb{R} )\to {\mathcal {C}}^{1}(S^{1},\mathbb{R} )$.

Then recall the definition of differentiability in terms of uniform linear approximation and see whether it can be satisfied at any base point $(\tau ,u)$.

YOUR SOLUTION WANTS TO BE HERE

local charts

Verify that ${\mathcal {B}}$ has local Banach manifold charts modeled on $E:=\{\xi \in {\mathcal {C}}^{1}(S^{1},\mathbb{R} )\,|\,\xi (0)=0\}$. To set up a chart near a given point, pick a representative $[u_{0}]\in {\mathcal {B}}$ with ${\dot u}_{0}(0)\neq 0$ and consider the map

$\phi _{{u_{0}}}:\{\xi \in E\,|\,\|\xi \|_{{{\mathcal {C}}^{1}}}<\epsilon \}\to {\mathcal {B}},\qquad \xi \mapsto [u_{0}+\xi ].$

YOUR SOLUTION WANTS TO BE HERE

transition maps

For $[u_{0}]=[u_{1}]\in {\mathcal {B}}$ with representatives $u_{0}\neq u_{1}$ but ${\dot u}_{i}(0)\neq 0$ determine the transition map $\phi _{{u_{1}}}^{{-1}}\circ \phi _{{u_{0}}}$.

Then find an example of a point and direction in which this transition map is not differentiable.

YOUR SOLUTION WANTS TO BE HERE

alternative Banach norms

Explain why working with ${\mathcal {C}}^{k}$, H\"older, or Sobolev spaces $W^{{k,p}}$ does not resolve these differentiabliity issues. Possibly come up with other ideas for a Banach manifold structure on ${\mathcal {B}}$.

YOUR SOLUTION WANTS TO BE HERE

reparametrization on Riemann surfaces

Explore the same questions for a space of nonconstant functions $u:\Sigma \to \mathbb{R}$ on a Riemann surface $(\Sigma ,j)$ modulo the reparametrization action $(\psi ,u)\mapsto u\circ \psi$ of a nontrivial automorphism group ${{\rm {Aut}}}(\Sigma ,j)=\{\psi :\Sigma \to \Sigma \,|\,\psi ^{*}j=j\}$ (e.g. $\Sigma =S^{2}{\text{or}}\;T^{2}$).

YOUR SOLUTION WANTS TO BE HERE

scale smoothness of the shift map

Show that the shift action $\sigma$ is a scale-smooth map by the following steps:

a) find a scale-Banach space ${\mathbb {E}}=(E_{\ell })_{{\ell \geq 0}}$ with $E_{0}=C^{1}(S^{1},{\mathbb R}^{2})$

b) show that 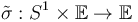${\tilde \sigma }:S^{1}\times {\mathbb {E}}\to {\mathbb {E}}$ is $sc^{1}$ and calculate $T\sigma$

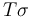
c) iteratively show that $\sigma$ is $sc^{k}$ for all k

Note: The actual map $\sigma$ above is a restriction of the map ${\tilde \sigma }$ to the subset ${\mathcal {C}}_{{{\rm {id}}}}^{1}\subset {\mathbb {E}}$ given by embedding $S^{1}=\{|z|=1\}\subset \mathbb{R} ^{2}$.