Ambient space
For the construction of a general 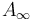 -composition map we are given
-composition map we are given  Lagrangians
Lagrangians  and a fixed autonomous Hamiltonian function
and a fixed autonomous Hamiltonian function  for each pair
for each pair  whose time-1 flow provides transverse intersections
whose time-1 flow provides transverse intersections  .
To simplify notation for consecutive Lagrangians in the list, we index it cyclically by
.
To simplify notation for consecutive Lagrangians in the list, we index it cyclically by  and abbreviate
and abbreviate  so that we have
so that we have  whenever
whenever  , and in particular
, and in particular  unless
unless  .
Now, given generators
.
Now, given generators 

 of these morphism spaces, we construct the Gromov-compactified moduli space of generalized pseudoholomorphic polygons by combining the two special cases above with sphere bubble trees,
of these morphism spaces, we construct the Gromov-compactified moduli space of generalized pseudoholomorphic polygons by combining the two special cases above with sphere bubble trees,

where
1. 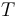 is an ordered tree with sets of vertices
is an ordered tree with sets of vertices  and edges
and edges  ,
,
equipped with orientations towards the root, orderings of incoming edges, and a partition into main and critical (leaf and root) vertices as follows:
2. The tree structure induces tuples of Lagrangians 
that label the boundary components of domains in overall counter-clockwise order  as follows:
as follows:
3.  is a tuple of generalized Morse trajectories
is a tuple of generalized Morse trajectories
in the following compactified Morse trajectory spaces:
4.  is a tuple of boundary points
is a tuple of boundary points
that correspond to the edges of 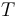 , are ordered counter-clockwise, and associate complex domains
, are ordered counter-clockwise, and associate complex domains  to the vertices as follows:
to the vertices as follows:
5.  is a tuple of sphere bubble tree attaching points for each main vertex
is a tuple of sphere bubble tree attaching points for each main vertex  , given by an unordered subset
, given by an unordered subset  of the interior of the domain.
of the interior of the domain.
6.  is a tuple of sphere bubble trees
is a tuple of sphere bubble trees  indexed by the disjoint union
indexed by the disjoint union  of sphere bubble tree attaching points.
of sphere bubble tree attaching points.
7.  is a tuple of pseudoholomorphic maps for each main vertex,
is a tuple of pseudoholomorphic maps for each main vertex,
that is each  is labeled by a smooth map
is labeled by a smooth map  satisfying Cauchy-Riemann equation, Lagrangian boundary conditions, finite energy, and matching conditions as follows:
satisfying Cauchy-Riemann equation, Lagrangian boundary conditions, finite energy, and matching conditions as follows:
8. The generalized pseudoholomorphic polygon is stable
in the sense that
Finally, two generalized pseudoholomorphic polygons are equivalent  if
if
there is a tree isomorphism  and a tuple of disk biholomorphisms
and a tuple of disk biholomorphisms  which preserve the tree, Morse trajectories, marked points, and pseudoholomorphic curves in the sense that
which preserve the tree, Morse trajectories, marked points, and pseudoholomorphic curves in the sense that
Warning: Our directional conventions differ somewhat from [Seidel book] and [J.Li thesis] as follows:
If no Hamiltonian perturbations are involved in the construction of a moduli space of generalized pseudoholomorphic polygons - i.e. if for each pair  the Lagrangians are either identical
the Lagrangians are either identical  or transverse
or transverse  - then the symplectic area function on the moduli space is defined by
- then the symplectic area function on the moduli space is defined by
![\omega :\overline {\mathcal {M}}(x_{0};x_{1},\ldots ,x_{d})\to \mathbb{R} ,\quad b={\bigl [}T,\underline {\gamma },\underline {z},\underline {w},\underline {\beta },\underline {u}{\bigr ]}\mapsto \omega (b):=\sum _{{v\in V^{m}}}\textstyle \int _{{\Sigma _{v}}}u_{v}^{*}\omega \;+\;\sum _{{\beta _{w}\in \underline {\beta }}}\omega (\beta _{w})=\langle [\omega ],[b]\rangle ,](/images/math/a/3/1/a3115e83f279a76a70eaf2f248d977cd.png)
which - since  only depends on the total homology class of the generalized polygon
only depends on the total homology class of the generalized polygon
![[b]:=\sum _{{v\in V}}(\overline {u}_{v})_{*}[D]+\sum _{{\beta _{w}\in \underline {\beta }}}[\beta _{w}]\;\in \;H_{2}(M;L_{0}\cup L_{1}\ldots \cup L_{d}).](/images/math/3/2/1/321a107845d6cd857b754c4884454f7a.png)
Here  is defined by unique continuous continuation to the punctures
is defined by unique continuous continuation to the punctures  at which
at which  or
or  .
.
Differential Geometric TODO:
In the presence of Hamiltonian perturbations, the definition of the symplectic area function needs to be adjusted to match with the symplectic action functional on the Floer complexes and satisfy some properties (which also need to be proven in the unperturbed case):
Sphere bubble trees
The sphere bubble trees that are relevant to the compactification of the moduli spaces of pseudoholomorphic polygons are genus zero stable maps with one marked point, as described in e.g. [Chapter 5, McDuff-Salamon].
For a fixed almost complex structure  , we can use the combinatorial simplification of working with a single marked point to construct the moduli space of sphere bubble trees as
, we can use the combinatorial simplification of working with a single marked point to construct the moduli space of sphere bubble trees as

where
1. 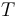 is a tree with sets of vertices
is a tree with sets of vertices  and edges
and edges  , and a distinguished root vertex
, and a distinguished root vertex  , which we use to orient all edges towards the root.
, which we use to orient all edges towards the root.
2.  is a tuple of marked points on the spherical domains
is a tuple of marked points on the spherical domains  ,
,
indexed by the edges of 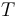 , and including a special root marked point as follows:
, and including a special root marked point as follows:
3.  is a tuple of pseudoholomorphic spheres for each vertex,
is a tuple of pseudoholomorphic spheres for each vertex,
that is each  is labeled by a smooth map
is labeled by a smooth map  satisfying
Cauchy-Riemann equation, finite energy, and matching conditions as follows:
satisfying
Cauchy-Riemann equation, finite energy, and matching conditions as follows:
4. The sphere bubble tree is stable
in the sense that
Finally, two sphere bubble trees are equivalent  if
if
there is a tree isomorphism  and a tuple of sphere biholomorphisms
and a tuple of sphere biholomorphisms  which preserve the tree, marked points, and pseudoholomorphic curves in the sense that
which preserve the tree, marked points, and pseudoholomorphic curves in the sense that
 are oriented towards the root vertex
are oriented towards the root vertex  (except for the root vertex which has no outgoing edge) and a (possibly empty) set of incoming edges
(except for the root vertex which has no outgoing edge) and a (possibly empty) set of incoming edges  .
.  . This induces a cyclic order on the set of all edges
. This induces a cyclic order on the set of all edges  adjacent to
adjacent to  , by setting
, by setting  , and we will denote consecutive edges in this order by
, and we will denote consecutive edges in this order by  . In particular this yields
. In particular this yields  .
.  into the sets of main vertices
into the sets of main vertices  and critical vertices
and critical vertices  . The latter is ordered to start with the root
. The latter is ordered to start with the root  and then contains d leaves
and then contains d leaves  of the tree, with order induced by the orientation and order of the edges.
of the tree, with order induced by the orientation and order of the edges. has a single edge
has a single edge  , and this attaches to a main vertex
, and this attaches to a main vertex  and
and  between its two critical vertices
between its two critical vertices  .
.  is a cyclic sequence of Lagrangians
is a cyclic sequence of Lagrangians  indexed by the adjacent edges
indexed by the adjacent edges  (which will become the boundary condition on
(which will become the boundary condition on  ).
). the Lagrangian labels satisfy a matching condition as follows:
the Lagrangian labels satisfy a matching condition as follows:
 requires
requires  .
. requires
requires  .
. requires
requires  and
and  .
. from a critical leaf
from a critical leaf  is labeled by a half-infinite Morse trajectory
is labeled by a half-infinite Morse trajectory  if
if  , resp. by the constant
, resp. by the constant  in the discrete space
in the discrete space  if
if  attaches to a main vertex
attaches to a main vertex  if
if  in the discrete space
in the discrete space  if
if  .
. between critical vertices is labeled by an infinite Morse trajectory
between critical vertices is labeled by an infinite Morse trajectory  (this occurs only for
(this occurs only for  and the tree with one edge
and the tree with one edge  between main vertices
between main vertices  is labeled by a finite or infinite Morse trajectory
is labeled by a finite or infinite Morse trajectory  in case
in case  , resp. by a constant
, resp. by a constant  in the discrete space
in the discrete space  in case
in case  . (Recall the matching condition
. (Recall the matching condition  and
and  from 2.)
from 2.) pairwise disjoint marked points
pairwise disjoint marked points  on the boundary of a disk.
on the boundary of a disk.  of the edges corresponds to a counter-clockwise order of the marked points
of the edges corresponds to a counter-clockwise order of the marked points  .
. and
and  by the edges
by the edges  for which
for which  partition the boundary into
partition the boundary into  such that the closure of each component
such that the closure of each component  .
.
 is a vector-field-valued 1-form on
is a vector-field-valued 1-form on  that is chosen compatibly with the fixed Hamiltonian perturbations as follows:
that is chosen compatibly with the fixed Hamiltonian perturbations as follows:
![\iota _{e}^{v}:[0,\infty )\times [0,1]\hookrightarrow \Sigma ^{v}](/images/math/d/1/1/d1100c1e7510c1014aac509f6b8fd6de.png) near each puncture
near each puncture  .
.
 forces the vector-field-valued 1-form on
forces the vector-field-valued 1-form on ![\Sigma ^{v}\simeq \mathbb{R} \times [0,1]](/images/math/5/6/a/56a3486ce4c38533929719c2fae3d318.png) in case
in case  to be
to be  -invariant,
-invariant,  if
if  are the Lagrangian labels for the boundary components
are the Lagrangian labels for the boundary components  .
.
 in case
in case  in case
in case  should be cut off to vanish outside of the thin parts of the domains
should be cut off to vanish outside of the thin parts of the domains  . However, there may be thin parts of a surface
. However, there may be thin parts of a surface  , and the surfaces
, and the surfaces  are replaced by a single
are replaced by a single  for
for  . Compatibility with gluing requires that the Hamiltonian perturbation on these glued surfaces is also given by a
. Compatibility with gluing requires that the Hamiltonian perturbation on these glued surfaces is also given by a  (in which the two perturbations
(in which the two perturbations  agree and hence can be matched over a long neck
agree and hence can be matched over a long neck ![[-R,R]\times [0,1]\subset \Sigma _{{v\#w}}](/images/math/7/c/1/7c10420071147771af7f4f746f0fc075.png) ).
).
 ; more precisely this requires
; more precisely this requires  for each adjacent edge
for each adjacent edge  .
. .
.  for each main vertex
for each main vertex  .
. near each puncture
near each puncture  associated to the edge
associated to the edge  , that is
, that is  for
for  .
.  , the map
, the map ![u_{e}^{v}:=(\iota _{e}^{v})^{*}u_{v}:(-\infty ,0)\times [0,1]\to M](/images/math/6/3/7/63792330def3bf1b30267f5afb4d519f.png) has a uniform limit
has a uniform limit  for some
for some  , and this limit intersection point is required to match with the value of the constant 'Morse trajectory'
, and this limit intersection point is required to match with the value of the constant 'Morse trajectory'  associated to the edge
associated to the edge  .
.  has enough special points to have trivial isotropy, that is the number of boundary marked points
has enough special points to have trivial isotropy, that is the number of boundary marked points  plus twice the number of interior marked points
plus twice the number of interior marked points  is at least 3.
is at least 3.
 preserves the tree structure and order of edges;
preserves the tree structure and order of edges;  for every
for every  ;
; for every
for every  for every
for every  for every
for every  for every
for every 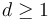 outgoing striplike ends.
Since we also insist on ordering the marked points counter-clockwise on the boundary of the disk, we then have to work with positive striplike ends
outgoing striplike ends.
Since we also insist on ordering the marked points counter-clockwise on the boundary of the disk, we then have to work with positive striplike ends ![[0,\infty )\times [0,1]\hookrightarrow \Sigma ^{v}](/images/math/b/e/c/bec1bfe842c6b1916c804663467dcec7.png) near each marked point
near each marked point  to make sure that the boundary components are labeled in order:
to make sure that the boundary components are labeled in order:  with
with  , and
, and  with
with  .
Analogously, a negative striplike end
.
Analogously, a negative striplike end ![(-\infty ,0]\times [0,1]\hookrightarrow \Sigma ^{v}](/images/math/c/7/3/c732b45e4625a7ffd19f60df1348fade.png) near the marked point
near the marked point  for the outgoing edge labels
for the outgoing edge labels  and
and  .
.
 the output of the differential
the output of the differential  includes a sum over (amongst other more complicated trees) pseudoholomorphic strips
includes a sum over (amongst other more complicated trees) pseudoholomorphic strips ![b=[u:\mathbb{R} \times [0,1]\to M]](/images/math/d/9/6/d96a1d9a44f24bab4af54724bdfa170d.png) with fixed positive limit
with fixed positive limit  .
.
 is invariant under deformations with fixed limits (used in proof of A-infty relations and invariance);
is invariant under deformations with fixed limits (used in proof of A-infty relations and invariance); to be of positive symplectic area, or constant strips/disks for zero symplectic area;
to be of positive symplectic area, or constant strips/disks for zero symplectic area; the tuple of mutually disjoint marked points
the tuple of mutually disjoint marked points  is indexed by the edges
is indexed by the edges  adjacent to
adjacent to  the tuple of mutually disjoint marked points
the tuple of mutually disjoint marked points  is also indexed by the edges adjacent to
is also indexed by the edges adjacent to  .
. , can also be denoted as
, can also be denoted as  .
. .
. for each edge
for each edge  . Here the marked point
. Here the marked point  of the root vertex; in other words the root vertex can be constant with just two adjacent edges.
of the root vertex; in other words the root vertex can be constant with just two adjacent edges.
 ;
;  and
and